Discrete Mathematics

It is designed swipe based for engineering student of all streams to learn discrete mathematics. It almost cover all important topics which are covered chapter wise.

Chapter 1 Set Theory, Relation, Function, Theorem Proving Techniques

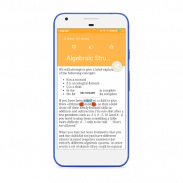
1. Set Theory

2. countable and uncountable sets

3. Venn Diagrams

4. proofs of some general identities on sets Relation in Venn Diagrams

5. types of relation

6. composition of relations

7. Equivalence relation

8. Partial ordering relation

9. one-to-one Function

10. into and onto function

11. Inverse Functions

12. Pigeonhole Principle

Chapter 2 Algebraic Structures

1. Algebraic structures

2. Abelian group

3. Subgroups

4. cyclic group

5. Homomorphism and isomorphism of Groups

6. Rings and Fields

Chapter 3 Propositional Logic

1. Proposition

2. Conditional Statements

3. Truth Tables of Compound Propositions

4. Logic and Bit Operations

5. PROPOSITIONAL EQUIVALENCES

6. Logical Equivalences

7. Constructing New Logical Equivalences

8. Predicates

9. Quantifiers

10. Infinite States and Infinite State Transitions

11. Finite state machines as language recognizers

Chapter 4 Graph Theory

1. Introduction of graphs

2. Basic Terms of Graph Theory

3. Planer graphs

4. multigraph

5. isomorphic Graph

6. paths, cycles, trails, and circuits

7. Shortest paths

8. Eulerian and Hamiltonian paths and circuits

9. Graph coloring

10. chromatic number

11. Homomorphism and isomorphism of Groups

Chapter 5 Posets, Hasse Diagram and Lattices

1. Posets, Hasse Diagram

2. ordered set

3. Hasse diagrams

4. isomorphic ordered set

5. well ordered set

6. properties of Lattices

7. bounded and complemented lattices

8. Combinatorics

9. Permutation and combination

10. Binomial Theorem

11. Introduction to Recurrence Relation and Recursive algorithms

12. Linear recurrence relations with constant coefficients

13. Homogeneous solutions

</div> <div jsname="WJz9Hc" style="display:none">Het is ontworpen op basis van swipe voor engineering student van alle stromen naar discrete wiskunde leren. Bedekken bijna alle belangrijke onderwerpen die onder hoofdstuk wijs.

Hoofdstuk 1 Set Theory, relatie, functie, Theorem Proving Technieken

1. Stel Theorie

2. telbare en ontelbare sets

3. Venn diagrammen

4. bewijzen van enkele algemene identiteiten op de sets Relation in Venn-diagrammen

5. soorten relatie

6. samengestelde relatie

7. Gelijkwaardigheid relatie

8. Gedeeltelijke bestellen relatie

9. één-op-één functie

10. in en op de functie

11. Inverse Functies

12. Pigeonhole Principe

Hoofdstuk 2 Algebraïsche Structuren

1. Algebraïsche structuren

2. Abelse groep

3. subgroepen

4. cyclische groep

5. homomorfisme en isomorfisme van groepen

6. Ringen en Velden

Hoofdstuk 3 propositielogica

1. Proposition

2. Voorwaardelijke Verklaringen

3. Waarheid tabellen van Compound Stellingen

4. Logica en Bit Operations

5. PROPOSITIONELE Gelijkwaardigheid

6. Logische Gelijkwaardigheid

7. aanleg van nieuwe Logische Gelijkwaardigheid

8. Predikaten

9. Quantifiers

10. Infinite Staten en Infinite State Transitions

11. Eindige state machines als taal recognizers

Hoofdstuk 4 Grafentheorie

1. Inleiding van de grafieken

2. Basis Voorwaarden Grafentheorie

3. Planer grafieken

4. Multigraph

5. isomorf Grafiek

6. paden, cycli, paden en circuits

7. Kortste paden

8. Euleriaanse en Hamiltoniaanse paden en circuits

9. Graafkleuring

10. chromatische nummer

11. homomorfisme en isomorfisme van groepen

Hoofdstuk 5 Posets, Hasse Diagram en Lattice

1. Posets, Hasse Diagram

2. geordende set

3. Hasse diagrammen

4. isomorf geordende set

5. goed geordende set

6. eigenschappen van Lattice

7. begrensd en aangevuld roosters

8. Combinatoriek

9. Permutatie en combinatie

10. Binomiale Stelling

11. Inleiding tot Relation en Recursieve algoritmen herhaling

12. Linear herhaling relaties met constante coëfficiënten

13. Homogene oplossingen</div> <div class="show-more-end">